Pentomino

A pentomino is a polyform of the polyomino class consisting of a geometric figure composed of five squares joined by their sides.

There are twelve different pentominoes, which are named with different letters of the alphabet. 

Pentominoes obtained from others by axial symmetry or by rotation do not count as a different pentomino. 

If we consider the pentominoes obtained by axial symmetry as different pentominoes, we have a total of 18. 

The so-called T, V, I, X, U, and W pentominoes form pentominoes by axial symmetry, which can also be obtained by rotation. 

It is interesting to note the different variations that can be obtained:

This set offers the possibility of merging the infinite possibilities of Lego with the thousands of puzzle options available with pentominoes.

The figures have been created respecting the dimensions and proportions of the pentaminos.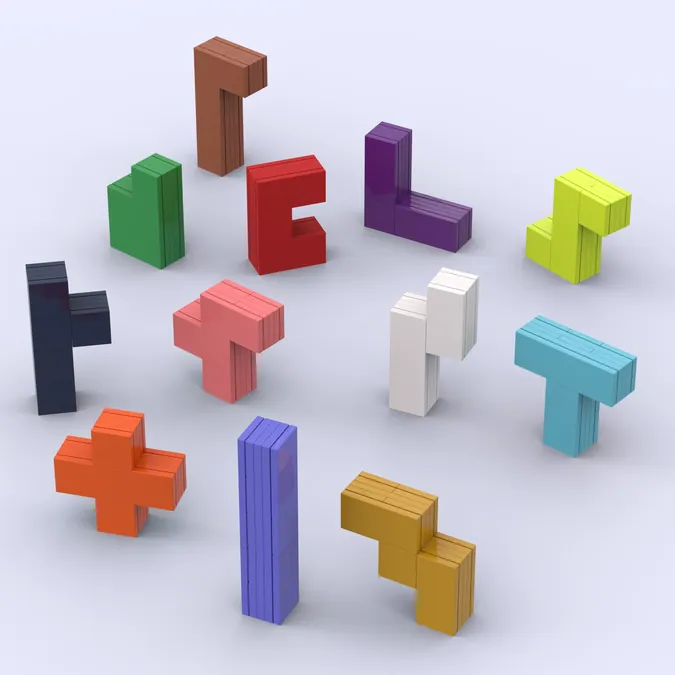

The set is completed with a specific number of pieces (243) to build the pentominoes and the frames of both the 2D and 3D layouts.

You have an example of 2D (in this idea its possible to build 4 different layouts)

and another of 3D (its possible to build 3 different layouts).

A 2D pentomino puzzle consists of filling a rectangle with the 12 different pentominoes, leaving no empty spaces or overlapping squares. Each of the 12 pentominoes occupies an area of ​​5 squares, so the rectangle must have a surface area of ​​60 squares. The possible dimensions are 6×10, 5×12, 4×15, and 3×20. It does not take a skilled player long to find a valid solution.

The 6×10 rectangle has exactly 2339 solutions, excluding variations obtained by rotation or symmetry of the entire rectangle, but including variations applied to a subset of pentominoes (sometimes this makes it easier to find other solutions).

The 5x12 rectangle has 1010 possible solutions, the 4x15 rectangle has 368 solutions, and the 3x20 rectangle has only 2.

A 3D pentomino puzzle consists of filling a three-dimensional box with the 12 pentominoes, without overlapping or leaving gaps. Each of the 12 pentominoes will be made up of 5 cubes, which will have the same shape as the 2-dimensional ones, but with volume. Obviously, the box must have a volume of 60 units and can have dimensions of 2x3x10, 2x5x6, or 3x4x5.

As if all this weren't enough, it's also possible to create a considerable number of shape puzzles (in the picture an ostrich), as well as the shapes of each and every piece of the pentamino. Throughout the updates, I'll be introducing many of the possible design possibilities.